MTU Department of Mathematics
Mathematics
Summer 2023
Publications
- J.P. McCarthy, Arch. Math. 121, 211–224 (2023). https://doi.org/10.1007/s00013-023-01883-w
Abstract. Using a suitably noncommutative flat matrix model, it is shown that the quantum permutation group has free orbitals: that is, a monomial in the generators of the algebra of functions can be zero for trivial reasons only. It is shown that any strictly intermediate quantum subgroup between the classical and quantum permutation groups must have free three-orbitals. This is used to give explicit formulae for the Haar state on degree four monomials that hold for such intermediate quantum subgroups as well as the quantum permutation group itself.
Presentations
- J.P. McCarthy, A quantum Frucht theorem, same talk given to Non local games seminar and C*-Days in Prague in May 2023.
- J.P. McCarthy, Another look at idempotent states (on quantum permutation groups), talk given to Quantum Groups and Interactions workshop in Glasgow, May 2023.
Winter 2022
PRESENTATIONS
- J.P. McCarthy, The Kawada-Ito theorem for quantum groups, UCC BRCI mini-Symposium on Noncommutative Probability & Quantum Information, October 2022.
Spring 2022
Publications
- Shane O'Rourke, Affine structures, wreath products and free affine actions on linear non-archimedean trees, Journal of Lie Theory. 32 (2022), no. 1, 157-174.
PResentations
- J.P. McCarthy, The Frucht property in the quantum group setting, Quantum Group Seminar, January 2022.
Winter 2021
Publications
- J.P. McCarthy, A state-space approach to quantum permutations, Expositiones Mathematicae, 2021, ISSN 0723-0869, https://doi.org/10.1016/j.exmath.2021.12.003.
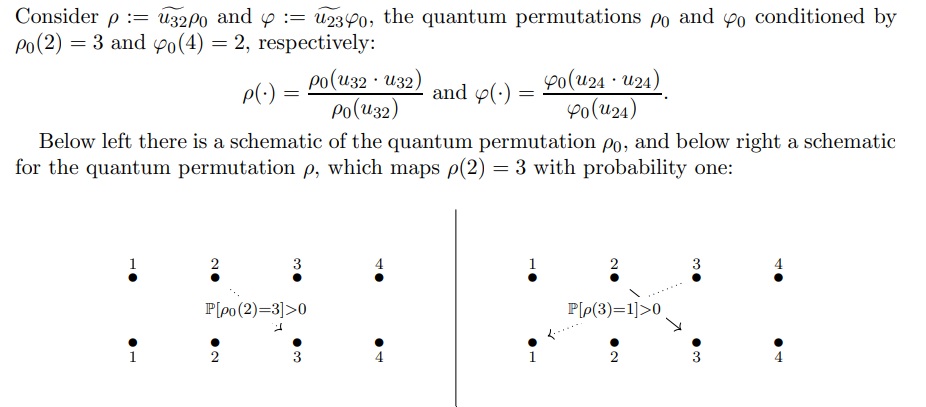
- Teo Banica and J.P. McCarthy, The Frucht property in the quantum group setting, Glasgow Mathematical Journal, 1-31, doi:10.1017/S0017089521000380
Spring 2021
Publications
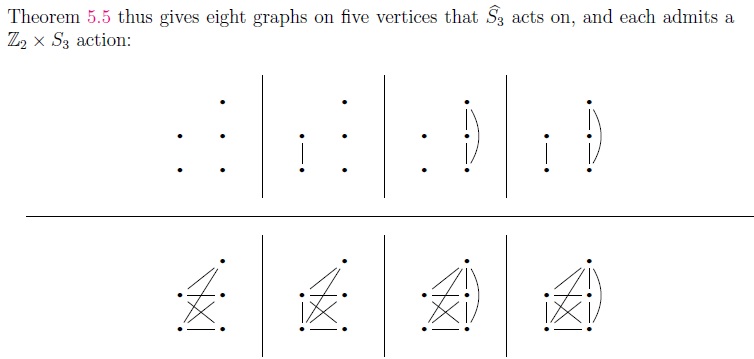
- J.P. McCarthy, The ergodic theorem for random walks on finite quantum groups, Communications in Algebra, DOI: 10.1080/00927872.2021.1908551
Winter 2020
Presentations
- J.P. McCarthy, Quantum Permuations Re-Born, Quantum Group Seminar (of Teo Banica, Universite de Cergy-Pontoise, Paris)
Winter 2019
Presentations
- J.P. McCarthy, The Ergodic Theorem for Random Walks: from Finite Groups, to Group Algebras, to Finite Quantum Groups, Munster Groups 2019, WIT.
- Shane O'Rourke, Free actions on metric lines. Talk at Groups and their Actions 2019 conference, Politecnica Slaska, Gliwice, Poland.
Spring 2019
PRESENTATIONS
- J.P. McCarthy, Some Unresolved and Unexplored Aspects of Random Walks on Quantum Groups, Séminaire d’Analyse Fonctionnelle, Laboratoire de Mathématiques de Besançon, Université de Franche-Comté
PUBLICATIONS
- J.P. McCarthy, Diaconis--Shahshahani Upper Bound Lemma for Finite Quantum Groups, Fourier Anal Appl, 25, 2463--2491, 2019.
Summer 2018
Presentations
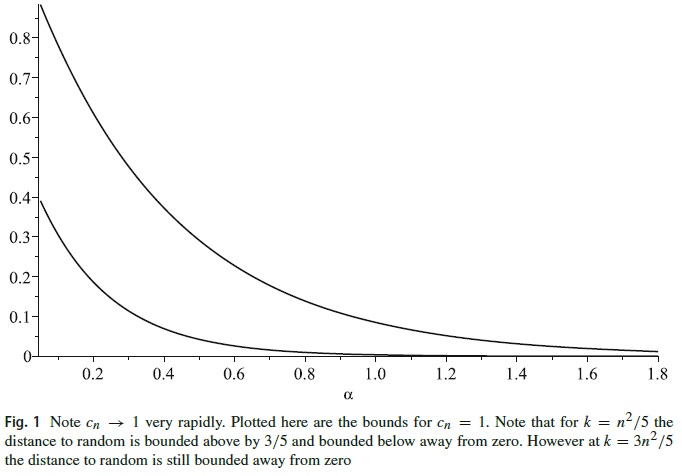
- J.P. McCarthy, The Diaconis-Shahshahani Upper Bound Lemma for Finite Quantum Groups, Irish Mathematical Society Meeting 2018, University College Dublin
Conference PRoceedings
-
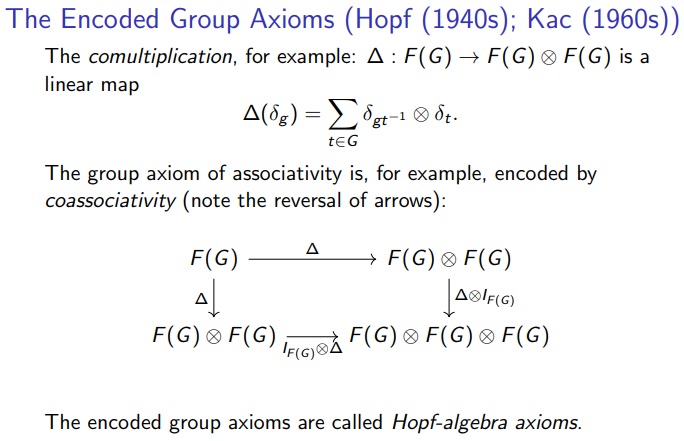
Marie Nicholson, The complexity of Tukey types and cofinal types, CiE (Computability in Europe)
Spring 2017
Presentations
- J.P. McCarthy, The Diaconis-Shahshahani Upper Bound Lemma for Finite Quantum Groups, Topological Quantum Groups an Harmonic Analysis Workshop, Seoul National University.
Summer 2016
Publications
-
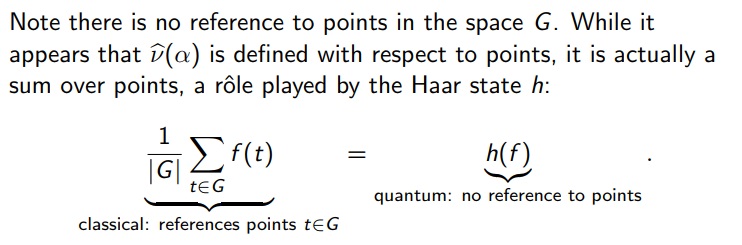
Shane O'Rourke, A combination theorem for affine tree-free groups Int. J. Alg. and Comput. 26 (2016), 1283-1321.
Presentations
- Shane O'Rourke, A combination theorem for affine tree-free groups. Talk at Geometric and Asymptotic Group Theory with Applications (GAGTA10) conference (Stevens Institute of Technology, New Jersey).
Spring 2016
Presentations
- J.P. McCarthy, CIT Department of Mathematics Spring Seminar Series, The Philosophy of Quantum Groups, January 2016.
- Shane O'Rourke, A combination theorem for affine tree-free groups. Invited speaker at Groups in Galway annual conference (NUI Galway).
Spring 2015
Publications
- Shane O'Rourke, A tree-free group that is not orderable Proc. Amer. Math. Soc. 143 (2015), 41-43.
