ERRORS

To understand the difficulties associated with the topic of transposition an error analysis was performed on students’ work. The deficiencies identified through the error analysis included: a lack of understanding of the equality sign and equivalent equations, incorrect use of inverse operations, mistakes expanding or simplifying equations, and inability to start a question. The types of errors identified by the error analysis are shown in the table below, along with examples of students’ work.

While all presented classes of deficiencies are major contributors to students’ struggles with rearranging equations, it is the lack of conceptual understanding that plays a major role here as without the deep understanding of what equations are, how they are formed and what the equality sign means other algebra skills become unproductive in the context of transposition. All non-conceptual deficiencies observed are algebraic in nature and constitute the basics necessary, but not sufficient, for manipulating equations and formulae.

Common errors	Class of maths defficiency	Some examples
Lack of understanding of the equality sign.	Conceptual understanding.
Incorrect use of inverse operations, including mistakes with signs.	Insufficient knowledge of the principles of transposition.
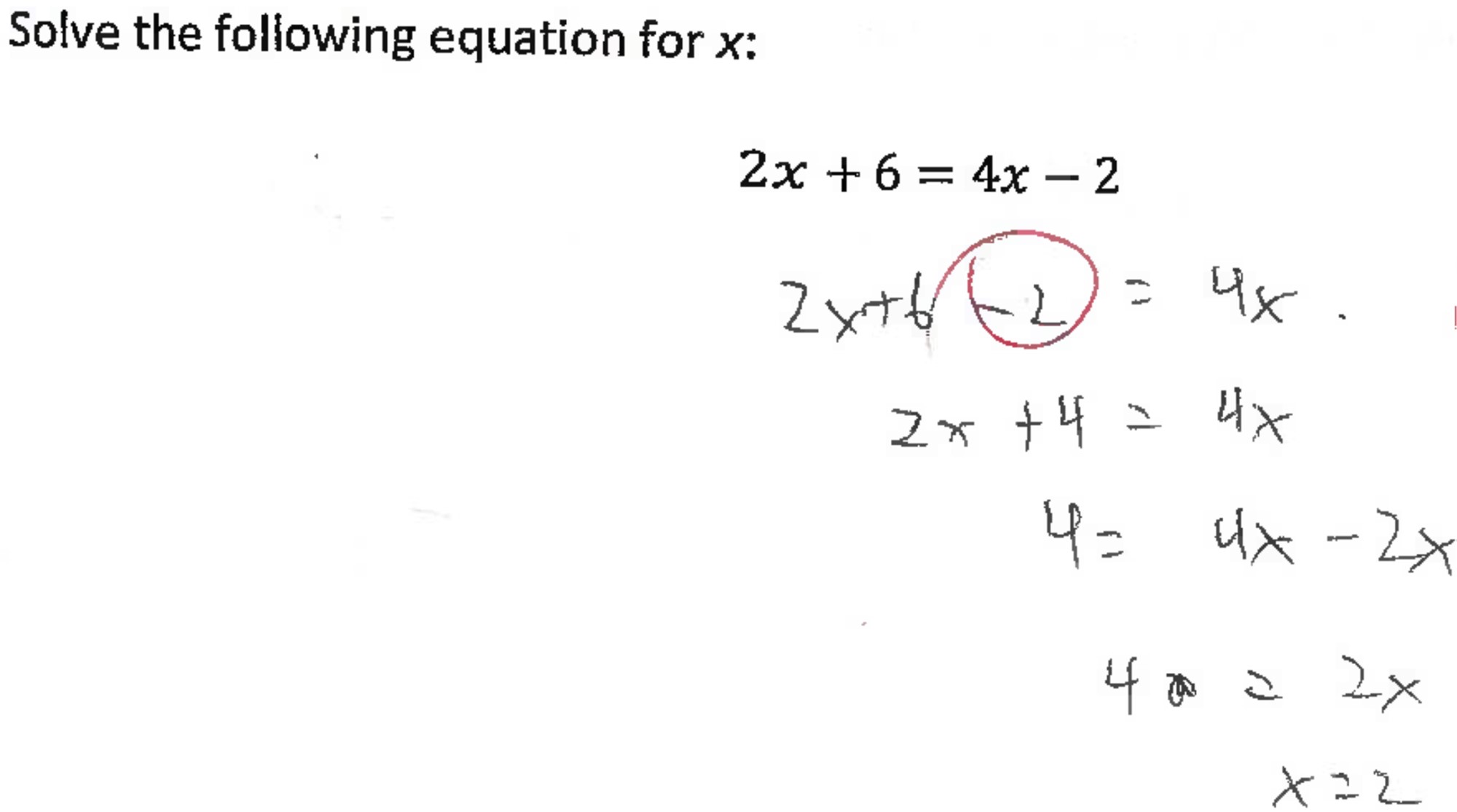Incorrect use of distributive law, factorising, fraction arithmetic and simplifying algebraic expressions.	Reprequisite algebra skills.
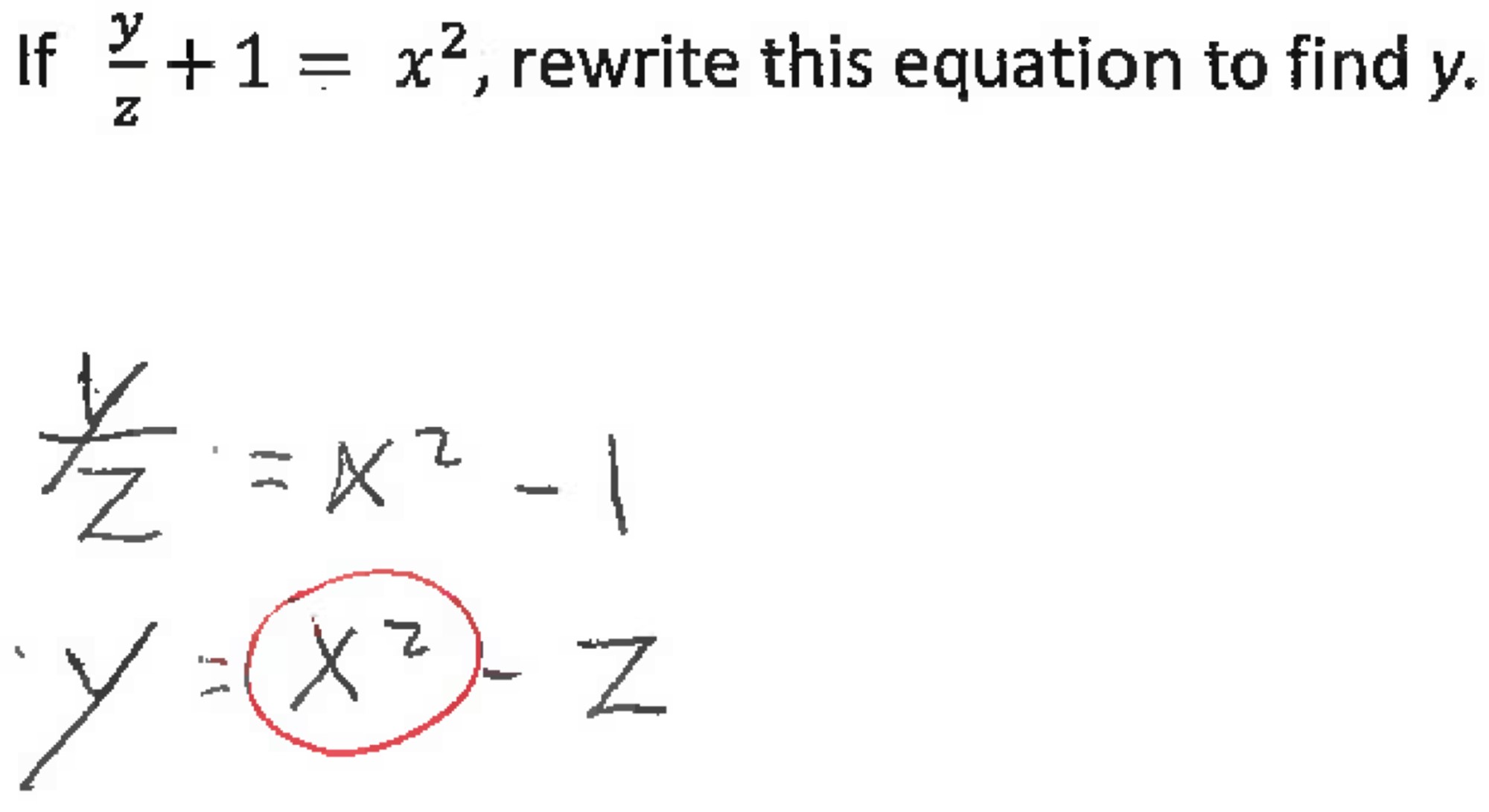Not knowing where to start.	Difficult to identify: possibly all of the deficiencies listed.

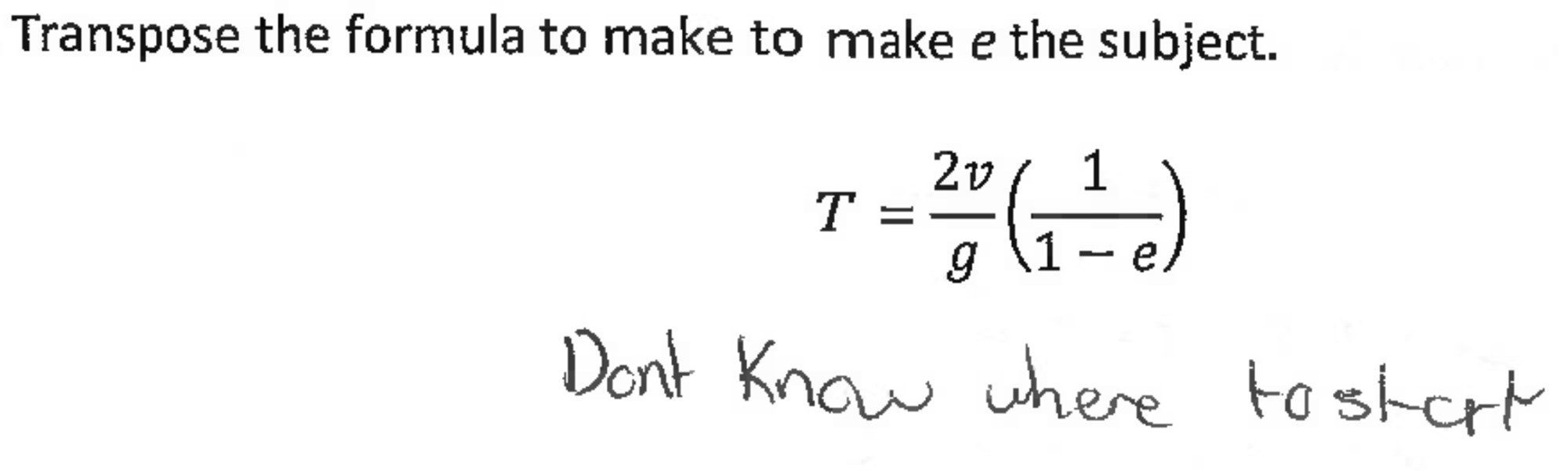MISCONCEPTIONS

Misconceptions most relevant to transposition may be roughly grouped into two classes: conceptual and algebraic.

Conceptual misconceptions manifest in:

• a lack of respect for the equality sign and hence mishandling the equations;
• incorrect use of inverse operations;
• illegal ‘moving’ of entities across an equation in a random fashion.

Algebraic misconceptions affecting transposition are mostly, but not limited to:

• misunderstanding and misuse of the distributive law;
• incorrect simplification of expressions (illegal ‘cancelling’);
• intuitive but wrong assumption about distributivity of exponents (squaring, taking roots etc.).

A schematic classification of misconceptions relevant to transposition and examples of typically encountered errors are given in the figure below.